137 Plus-or-Minus Groups of Magic Tori of Order 4, Linked by Plus-and-Minus Operations

Pandiagonal and semi-pandiagonal magic tori of order 4 connected by plus-and-minus links

Previous Studies

General information about the 255 magic tori of order 4, and about the 880 magic squares that those tori display, can be found in a previous article, published on the 15th June 2023, and entitled "440 Torus-Opposite Pairs of the 880 Frénicle Magic Squares of Order-4".

The 137 complete-torus, same-integer, plus-or-minus groups of magic tori of order 4 have been presented in a previous article, published on the 24th April 2024, and entitled "Plus or Minus Groups of Magic Tori of Order 4".

Further information about the magic tori can be found in the lists of references that are appended to the papers in each of the above-mentioned articles.

Searching for 2-or-more-integer plus-and-minus links between the 137 ± Groups of Magic Tori

As we are looking for connections between complete-torus, same-integer, plus-or-minus groups of magic tori, it seems logical to search for links with 2-or-more-integer plus-and-minus operations. The closest links between the tori will be those where only 4 out of the 16 numbers are changed, and these will be for example 3/4 & 1/4: (±0, ±1), or 3/4 & 1/4: (±0, ±2) etc., depending on the differences between the cell entries.

A variant of these links will be those where 12 out of the 16 numbers are changed, and these can be written, for example, as 3/4 & 1/4: (±1, ±0), or as 3/4 & 1/4: (±2, ±0) etc., depending on the differences between the cell entries. However, a change of only 1/4 of the cell entries seems less disruptive than a change of 3/4 of these, and we can therefore suppose that the previously-mentioned 3/4 & 1/4: (±0, ±1), or 3/4 & 1/4: (±0, ±2) are closer links.

Close links also include those where only 8 out of the 16 numbers are changed, and these will be, for example, 1/2 & 1/2: (±0, ±1), or 1/2 & 1/2: (±0, ±2) etc., depending on the differences between the torus cell entries.

In some cases 2-integer plus-and-minus operations cannot be found, but more-complex 3-integer links may exist, such as 1/2, 3/8 & 1/8: (±2, ±0, ±1) or 1/2, 1/4 & 1/4: (±4, ±0, ±8). 3-integer links like these are frequent between the paired complementary and self-complementary torus groups.

Sometimes even 3-integer plus-and-minus operations are unavailable, but there exist 4-integer links, such as 1/4, 1/4, 1/4, & 1/4: (±0, ±2, ±4, ±6), or 3/8, 1/4, 1/4 & 1/8: (±3, ±0, ±6, ±9), which, if not particularly close, show interesting patterns. Links like these are common within the paired complementary torus groups that are to be found in the pages that follow.

Some Precisions Concerning the Enclosed Presentation

Because of its A3 format, this enclosed PDF file can at first seem unwieldy, especially when it is displayed on small-screen mobile phones. However the large display size allows same-page illustrations of complete sets of ± groups, which can be comprised of up to 24 examples. Please bear in mind that, in order to reduce space requirements and facilitate reading, most of the 137 ± groups are represented by single magic square viewpoints of only one of their magic tori. But occasionally, a same group is represented more than once, so as to facilitate the comprehension of multiple links. To find our which other magic tori are within a ± group, please refer to the details given in the paper "Plus or Minus Groups of Magic Tori of Order 4", already mentioned above.

In order to simplify the annotation of the pages of the paper enclosed below, the links between the ± groups are written as ± operations. This is slightly ambiguous, as there must always be a same number of plusses as minuses of any particular integer. Here therefore, the ± sign in front of an integer will mean an equal number of plusses and minuses of that integer. This will become evident as soon as we compare any pair of magic square viewpoints which are connected by the links in question. For full details, please refer to the paper below.

Read more ...

Plus or Minus Groups of Magic Tori of Order 4

As the positive integer entries of normal magic tori, and of their displayed magic squares, are always arranged in same-sum orthogonal arrays, it would seem logical to compensate any additions or subtractions to those entries, by making corresponding subtractions or additions. How can we develop this simple observation?

Preliminary Definitions

A normal magic square is an N x N array of same-sum rows, columns and main diagonals. If the magic diagonal condition is not satisfied, the square is deemed to be semi-magic. The torus, upon which a magic or semi-magic square is displayed, can be visualised by joining the opposite edges of the magic or semi-magic square in question.

There are N x N square viewpoints on the surface of a torus of order N. A normal magic torus has N x N arrays of same-sum latitudes and longitudes, and at least one magic intersection of magic diagonals on its surface. In his paper "Conformal Tiling on a Torus" published by Bridges in 2011, John M. Sullivan shows that, on a square torus T1, 0, the diagonal grid lines “form (1, ±1) diagonals on the torus, each of which is a round (Villarceau) circle in space.”

Semi-magic tori can also have magic diagonals, but the latter can never produce the magic intersections required for magic squares, either because the magic diagonals are single or parallel, or because they do not intersect correctly. Additional information about magic and semi-magic tori, with further explanations of their diagonals, can be found in the references at the end of the enclosed paper.

In order 4, starting with any magic torus, and examining cases where half of the torus numbers are subjected to equal additions, and the other half are subjected to same-integer subtractions, we notice the following: From its initial state (which we can call 0), each magic square can be transformed by four plus or minus operations that will either produce alternative magic or semi-magic square viewpoints of the same torus, or square viewpoints of other essentially different magic or semi-magic tori. Here's a simple example of two transformations:

3 Magic Tori of Order 4 linked by by Complete-Torus, Same-Integer, Plus or Minus Operations

Complete-Torus, Same-Integer, Plus or Minus Groups in Order 4

Please note that, in order 4, the orthogonal totals of magic and semi-magic tori are always 34. Therefore, only the diagonal totals, which can vary, are announced here. These totals are interesting because of their symmetries, and in the example illustrated above, there are pairs of diagonals that always sum to 68 (twice the magic sum). Each of the 255 magic tori of order 4 is similarly examined, and the results are given in tables and observations at the end of the following paper: "Groups of Magic Tori of Order 4 Assembled by Complete-Torus, Same-Integer, Plus or Minus Operations"

Complete-Torus, Same-Integer, Plus or Minus Groups in Orders N > 4

The same method cannot always be applied to higher even orders. A quick look at some examples from even orders N = 6, N = 8, and N = 10 shows that certain magic tori have either no solutions whatsoever, or only one that can be used in a complete-torus same-integer plus or minus matrix to produce another orthogonally magic torus. In singly-even orders, even divisors with odd quotients have to be ruled out. Each case will need to be tested, and systematic computer checks will be necessary for these higher even orders.

An adaptation is of course required for odd orders, as their odd square totals do not have even integer divisors. But partial-torus same-integer divisions produce plus or minus solutions, and there are a variety of approaches.

The following paper, entitled "Examples of Partial Groups of Magic Tori of Orders N > 4 Assembled by Complete-Torus (or Near-Complete in Odd Orders) Same-Integer, Plus or Minus Operations" shows how the method used for order 4 gives some good results in higher orders:

Read more ...

440 Torus-Opposite Pairs of the 880 Frénicle Magic Squares of Order-4

Finding the torus-opposite pairs of magic squares in even-orders:

The following diagram, which illustrates the array of n² essentially-different square viewpoints of a basic magic torus of order-n, starting here with the 4x4 magic square that has the Frénicle index n° 1, shows how a torus-opposite magic square of order-4 can be identified:

Array of 16 essentially-different square viewpoints of a basic magic torus of order-4

This basic magic torus, now designated as type n° T4.05.1.01, can be seen to display a torus-opposite pair of Frénicle-indexed magic squares n° 1-458 (with Dudeney pattern VI), as well as 7 torus-opposite pairs of semi-magic squares that the discerning reader will easily be able to spot.

The relative positions of the numbers of torus-opposite squares can be expressed by a simple plus or minus vector. There are always two equal shortest paths towards the far side of the torus: These are, in toroidal directions, east or west along the latitudes, and in poloidal directions, north or south along the longitudes of the doubly-curved 2D surface. So, if the even-order is n, then:

v  =  ( ± n/2, ± n/2 )

"Tesseract Torus" by Tilman Piesk CC-BY-4.0
 https://commons.wikimedia.org/w/index.php?curid=101975795

Torus-opposite squares are not just limited to basic magic tori, as they also exist on pandiagonal, semi-pandiagonal, partially pandiagonal, and even semi-magic tori of even-orders. Therefore, for order-4, we can either say that there are 880 magic squares, or we can announce that there are 440 torus-opposite pairs of magic squares. Similarly, the 67,808 semi-magic squares of order-4 can be expressed as 33,904 torus-opposite pairs of semi-magic squares, etc.

Why torus-opposite pairs of magic squares cannot exist in odd-orders

A torus-opposite magic square always exists in even-orders because an even-order magic square has magic diagonals that produce a first magic intersection at a centre between numbers, and another magic intersection at a second centre between numbers on the far side of the torus (at the meeting point of the four corners of the first magic square viewpoint). However, in odd-orders, where a magic square has magic diagonals that produce a magic intersection over a number, then a sterile non-magic intersection always occurs between numbers on the far side of the torus (at the meeting point of the four corners of the initial magic square). This is why torus-opposite pairs of magic squares cannot exist in the odd-orders.

255 magic tori of order-4, listed by type, with details of the 440 torus-opposite pairs of the 880 Frénicle magic squares of order-4

The enumeration of the 255 magic tori of order-4 was first published in French on the 28th October 2011, before being translated into English on the 9th January 2012: "255 Fourth-Order Magic Tori, and 1 Third-Order Magic Torus." In this previous article, the corresponding 880 fourth-order magic squares were listed by their Frénicle index numbers, but not always illustrated. The intention of the enclosed paper is therefore to facilitate the understanding of the magic tori of order-4, by portraying each case.

Here, Frénicle index numbers continue to be used, as they have the double advantage of being both well known and commonly accepted for cross-reference purposes. But please also note, that now, in order to simplify the visualisation of the magic tori, their displayed magic squares are not systematically presented in Frénicle standard form. 

In the illustrations of the 255 magic tori of order-4, listed by type with the presentation of their magic squares, the latter are labelled from left to right with their Frénicle index number, followed by, in brackets, the Frénicle index number of their torus-opposite magic square, and finally by the Roman numeral of their Dudeney complementary number pattern. Therefore, for the magic square of order-4 with Frénicle index n° 1 (that forms a torus-opposite pair of magic squares with Frénicle index n° 458; both squares having the same Dudeney pattern VI), its label is 1 (458) VI.

To find the "255 magic tori of order-4, listed by type, with details of the 440 torus-opposite pairs of the 880 Frénicle magic squares of order-4" please consult the following PDF:

Read more ...

Polyomino Area Magic Tori

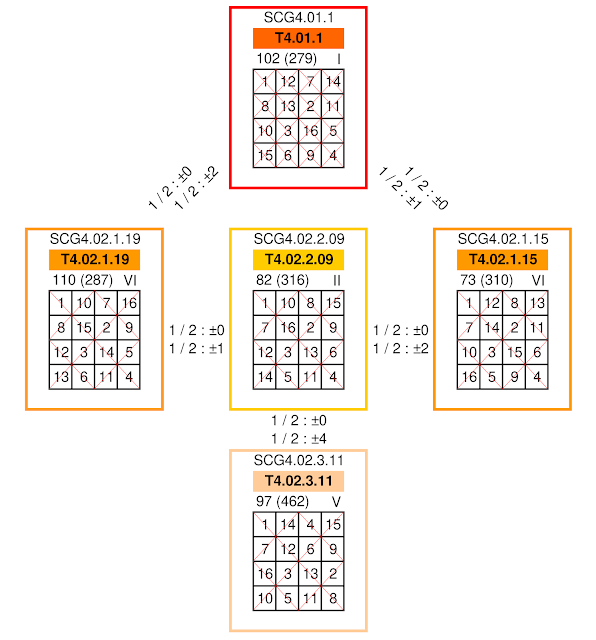
A magic torus can be found with any magic square, as has already been demonstrated in the article "From the Magic Square to the Magic Torus". In fact, there are n^2 essentially different semi-magic or magic squares, displayed by every magic torus of order-n. Particularly interesting to observe with pandiagonal (or panmagic) examples, a magic torus can easily be represented by repeating the number cells of one of its magic square viewpoints outside its limits. However, once we begin to look at area magic squares, it becomes much less evident to visualise and construct the corresponding area magic tori using repeatable area cells, especially when the latter have to be irregular quadrilaterals... The following illustration shows a sketch of an area magic torus of order-3 that I created back in January 2017. I call it a sketch because it may be necessary to use consecutive areas starting from 2 or from 3, should the construction of an area magic torus of order-3, using consecutive areas from 1 to 9, prove to be impossible. And while it can be seen that such a torus is theoretically constructible, many calculations will be necessary to ensure that the areas are accurate, and that the irregular quadrilateral cells can be assembled with precision:

At the time discouraged by the complications of such geometries, I decided to suspend the research of area magic tori. But since the invention of area magic squares, other authors have introduced some very interesting polyomino versions that open new perspectives: 

On the 20th May 2021, Morita Mizusumashi (盛田みずすまし @nosiika) tweeted a nice polyomino area magic square of order-3 constructed with 9 assemblies of 5 to 13 monominoes. On the 21st May 2021, Yoshiaki Araki (面積魔方陣がテセレーションみたいな件 @alytile) tweeted several order-4 and order-3 solutions in a polyomino area magic square thread. These included (amongst others) an order-4 example constructed with 16 assemblies of 5 to 20 dominoes. On the 24th May 2021, Yoshiaki Araki then tweeted an order-3 polyomino area magic square constructed using 9 assemblies of 1 to 9 same-shaped pentominoes! Edo Timmermans, the author of this beautiful square, had apparently been inspired by Yoshiaki Araki's previous posts! Since the 22nd June 2021, Inder Taneja has also published a paper entitled "Creative Magic Squares: Area Representations" in which he studies polyomino area magic using perfect square magic sums.

Intention and Definition

The intention of the present article is to explore the use of polyominoes for area magic torus construction, with the objective of facilitating the calculation and verification of the cell areas, while avoiding the geometric constraints of irregular quadrilateral assemblies. Here, it is useful to give a definition of a polyomino area magic torus:

1/ In the diagram of the torus, the entries of the cells of each column, row, and of at least two intersecting diagonals, will add up to the same magic sum. The intersecting magic diagonals can be offset or broken, as the area magic torus has a limitless surface, and can therefore display semi-magic square viewpoints.

2/ Each cell will have an area in proportion to its number. The different areas will be represented by tiling with same-shaped holeless polyominoes.

3/ The cells can be of any regular or irregular rectangular shape that results from their holeless tiling. 

4/ Depending on the order-n of the area magic torus, each cell will have continuous edge connections with contiguous cells (and these connections can be wrap-around, because the torus diagram represents a limitless curved surface).

5/ The vertex meeting points of four cells can only take place at four convex (i.e. 270° exterior  angled) vertices of each of the cells.

Polyomino Area Magic Tori (PAMT) of Order-3

Magic Torus index n° T3, of order-3. Magic sums = 15.
Please note that this is not a Polyomino Area Magic Torus,
but it is the Agrippa "Saturn" magic square, after a rotation of +90°, in Frénicle standard form.

Polyomino Area Magic Torus (PAMT) of order-3. Magic sums = 15. Tetrominoes.
Consecutively numbered areas 1 to 9, in an irregular rectangular shape of 180 units.

Polyomino Area Magic Torus (PAMT) of Order-3. Magic sums = 18. Trominoes.
Consecutively numbered areas 2 to 10, in an irregular rectangular shape of 162 units.

Polyomino Area Magic Torus (PAMT) of order-3. Magic sums = 24. Trominoes.
Consecutively numbered areas 4 to 12, in an oblong 12 ⋅ 18 = 216 units.

Polyomino Area Magic Torus (PAMT) of Order-3. Magic sums = 36. Trominoes Version 1.
Square 18 ⋅ 18 = 324 units.

Polyomino Area Magic Torus (PAMT) of Order-3. Magic sums = 36. Trominoes Version 2.
Square 18 ⋅ 18 = 324 units.

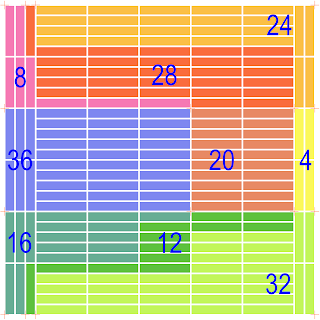Polyomino Area Magic Torus (PAMT) of Order-3. Magic sums = 60. Pentominoes Version 1.
Square 30 ⋅ 30 = 900 units.

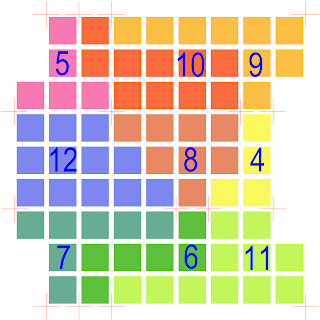Polyomino Area Magic Torus (PAMT) of order-3. Magic sums = 24. Monominoes.
Consecutively numbered areas 4 to 12, in an irregular rectangular shape of 72 units.

Polyomino Area Magic Torus (PAMT) of order-3. Magic sums = 27. Monominoes Version 1.
Consecutively numbered areas 5 to 13, in a square 9 ⋅ 9 = 81 units.

Polyomino Area Magic Torus (PAMT) of order-3. Magic sums = 27. Monominoes Version 2.
Consecutively numbered areas 5 to 13, in a square 9 ⋅ 9 = 81 units.

Polyomino Area Magic Tori (PAMT) of Order-4

Magic Torus index n° T4.198, of order-4. Magic sums = 34.
Please note that this is not a Polyomino Area Magic Torus,
but it is a pandiagonal torus represented by a pandiagonal square that has Frénicle index n° 107.

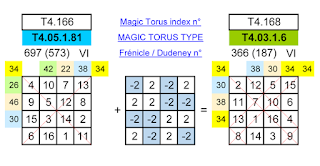
The pandiagonal torus above displays 16 Frénicle indexed magic squares n° 107, 109, 171, 204, 292, 294, 355, 396, 469, 532, 560, 621, 691, 744, 788, and 839. It is entirely covered by 16 sub-magic 2x2 squares. The torus is self-complementary and has the magic torus complementary number pattern I. The even-odd number pattern is P4.1. This torus is extra-magic with 16 extra-magic nodal intersections of 4 magic lines. It displays pandiagonal Dudeney I Nasik magic squares. It is classified with a Magic Torus index n° T4.198, and is of Magic Torus type n° T4.01.2. Also, when compared with its two pandiagonal torus cousins of order-4, the unique Magic Torus T4.198 of the Multiplicative Magic Torus MMT4.01.1 is distinguished by its total self-complementarity.

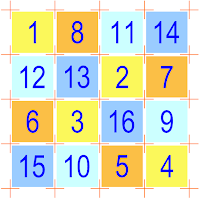
Pandiagonal Polyomino Area Magic Torus (PAMT) of order-4. Magic sums = 34. Pentominoes.
Index PAMT4.198, Version 1, Viewpoint 1/16, displaying Frénicle magic square index n° 107.
Consecutively numbered areas 1 to 16, in an oblong 34 ⋅ 20 = 680 units.

Pandiagonal Polyomino Area Magic Torus (PAMT) of order-4. Magic sums = 50. Dominoes.
Version 1, Viewpoint 1/16.
Consecutively numbered areas 5 to 20, in a square 20 ⋅ 20 = 400 units.

Pandiagonal Polyomino Area Magic Torus (PAMT) of Order-4. Sums = 50. Dominoes.
Version 1, Viewpoint 16/16.
Consecutively numbered areas 5 to 20, in an irregular rectangular shape of 400 units.

Polyomino Area Magic Tori (PAMT) of Order-5

Pandiagonal Torus type n° T5.01.00X of order-5. Magic sums = 65.
Please note that this is not a Polyomino Area Magic Torus.

This pandiagonal torus of order-5 displays 25 pandiagonal magic squares. It is a direct descendant of the T3 magic torus of order-3, as demonstrated in page 49 of "Magic Torus Coordinate and Vector Symmetries" (MTCVS). In "Extra-Magic Tori and Knight Move Magic Diagonals" it is shown to be an Extra-Magic Pandiagonal Torus Type T5.01 with 6 Knight Move Magic Diagonals. Note that when centred on the number 13, the magic square viewpoint becomes associative. The torus is classed under type n° T5.01.00X (provisional number), and is one of 144 pandiagonal or panmagic tori type 1 of order-5 that display 3,600 pandiagonal or panmagic squares. On page 72 of "Multiplicative Magic Tori" it is present within the type MMT5.01.00x.

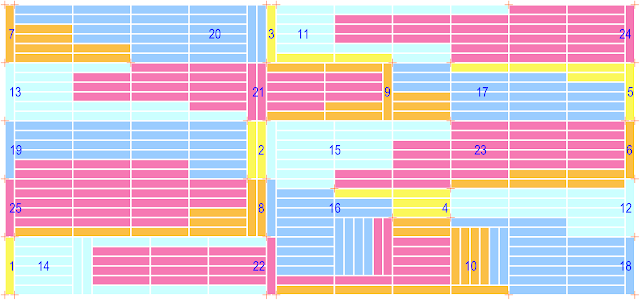Pandiagonal Polyomino Area Magic Torus (PAMT) of order-5. Magic sums = 65. Hexominoes.
Index PAMT5.01.00X, Version 1, Viewpoint 1/25.
Consecutively numbered areas 1 to 25, in an oblong 65 ⋅ 30 = 1950 units.

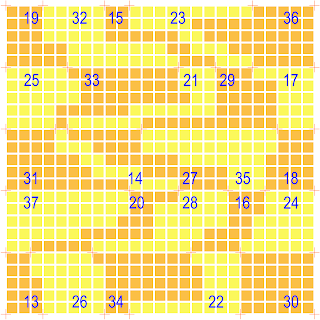Pandiagonal Polyomino Area Magic Torus (PAMT) of Order-5. Magic sums = 125. Monominoes.
Version 1, Viewpoint 1/25.
Consecutively numbered areas 13 to 37, in a square 25 ⋅ 25 = 625 units.

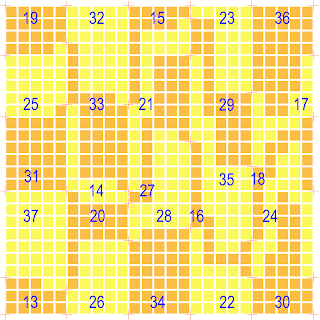Pandiagonal Polyomino Area Magic Torus (PAMT) of Order-5. Magic sums = 125. Monominoes.
Version 2, Viewpoint 1/25.
Consecutively numbered areas 13 to 37, in a square 25 ⋅ 25 = 625 units.

Polyomino Area Magic Tori (PAMT) of Order-6

Partially Pandiagonal Torus type n° T6 of order-6. Magic sums = 111.
Please note that this is not a Polyomino Area Magic Torus.

Harry White has kindly authorised me to use this order-6 magic square viewpoint. With a supplementary broken magic diagonal (24, 19, 31, 3, 5, 29), this partially pandiagonal torus displays 4 partially pandiagonal squares and 32 semi-magic squares. In "Extra-Magic Tori and Knight Move Magic Diagonals" it is shown to be an Extra-Magic Partially Pandiagonal Torus of Order-6 with 6 Knight Move Magic Diagonals. This is one of 2627518340149999905600 magic and semi-magic tori of order-6 (total deduced from findings by Artem Ripatti - see OEIS A271104 "Number of magic and semi-magic tori of order n composed of the numbers from 1 to n^2").

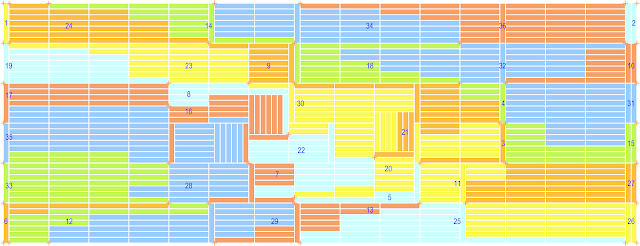Partially Pandiagonal Polyomino Area Magic Torus (PAMT) of order-6. Magic sums = 111.
Heptominoes, Version 1, Viewpoint 1/36.
Consecutively numbered areas 1 to 36, in an oblong 111 ⋅ 42 = 4662 units.

Partially Pandiagonal Polyomino Area Magic Torus (PAMT) of order-6. Sums = 147.
Dominoes, Version 1, Viewpoint 1/36.
Consecutively numbered areas 7 to 42, in a square 42 ⋅ 42 = 1764 units.

Observations

As they are the first of their kind, these Polyomino Area Magic Tori (PAMT) can most likely be improved: The examples illustrated above are all constructed with their cells aligned horizontally or vertically; and though it is convenient to do so, because it allows their representation as oblongs or squares, this method of constructing PAMT is not obligatory. Representations of PAMT that have irregular rectangular contours may well give better results, with less-elongated cells and simpler cell connections. 

While the use of polyominoes has the immense advantage of allowing the construction of area magic tori with easily quantifiable units, it also introduces the constraint of the tiling of the cells. It has been seen in the examples above that the PAMT can be represented as oblongs or as squares, while other irregular rectangular solutions also exist. A normal magic square of order-3 displays the numbers 1 to 9 and has a total of 45, which is not a perfect square. As the smallest addition to each of the nine numbers 1 to 9, in order to reach a perfect square total is four (45 + 9 ⋅ 4 = 81), this implies that when searching for a square PAMT with consecutive areas of 1 to 9, in theory the smallest polyominoes for this purpose will be pentominoes.

But to date, in the various shaped examples of PAMT shown above, the smallest cell area used to represent the area 1 is a tetromino, as this gives sufficient flexibility for the connections of a nine-cell PAMT of order-3 with consecutive areas of 1 to 9. Edo Timmermans has already constructed a Polyomino Area Magic Square of order-3 using pentominoes for the consecutive areas of 1 to 9, but it seems that such polyominoes cannot be used for the construction of a same-sized and shaped PAMT of order-3. Straight polyominoes are always used in the examples given above, as these facilitate long connections, but other polyomino shapes will in some cases be possible.

We should keep in mind that the PAMT are theoretical, in that, per se, they cannot tile a torus: As a consequence of Carl Friedrich Gauss's "Theorema Egregium", and because the Gaussian curvature of the torus is not always zero, there is no local isometry between the torus and a flat surface: We can't flatten a torus without distortion, which therefore makes a perfect map of that torus impossible. Although we can create conformal maps that preserve angles, these do not necessarily preserve lengths, and are not ideal for our purpose. And while two topological spheres are conformally equivalent, different topologies of tori can make these conformally distinct and lead to further mapping complications. For those wishing to know more, the paper by Professor John M. Sullivan, entitled "Conformal Tiling on a Torus", makes excellent reading.

Notwithstanding their theoreticality, the PAMT nevertheless offer an interesting field of research that transcends the complications of tiling doubly-curved torus surfaces, while suggesting interesting patterns for planar tiling: For those who are not convinced by 9-colour tiling, 2-colour pandiagonal tiling can also be a good choice for geeky living spaces:

Tiling with irregular rectangular shaped PAMT of order-3. Monominoes. S=24.

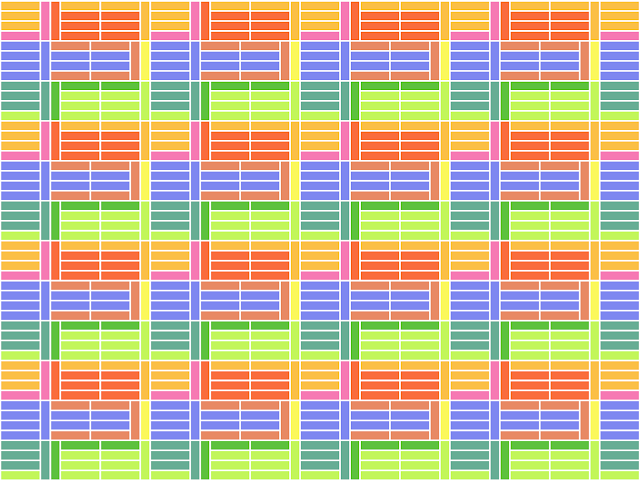Tiling with irregular rectangular shaped PAMT of order-3. Tetrominoes. S=15.

Tiling with irregular rectangular shaped PAMT of order-3. Trominoes. S=18.

Tiling with oblong PAMT of order-3. Trominoes. S=24.

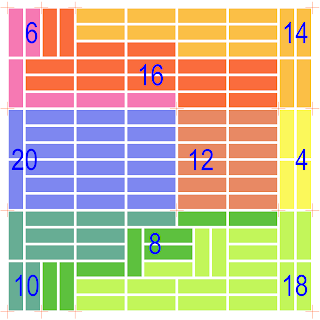
Tiling with oblong pandiagonal PAMT of order-4. Pentominoes. S=34.

Tiling with oblong pandiagonal PAMT of order-5. Hexominoes. S=65.

Tiling with square pandiagonal PAMT of order-5. Monominoes. S=125.

Tiling with oblong partially pandiagonal PAMT of order-6. Heptominoes. S=111.

Tiling with square partially pandiagonal PAMT of order-6. Dominoes. S=147.

There are still plenty of other interesting PAMT that remain to be found, and I hope you will authorise me to publish or relay your future discoveries and suggestions!

Read more ...

How Dürer's "Melencolia I" is a painful but liberating metamorphosis!

The title of this post may at first seem rather strange, especially when we know that the main subjects of these pages are "Magic Squares, Spheres and Tori." However, the famous "Melencolia I" engraving by Albrecht Dürer does depict, amongst other symbols, a magic square of order-4 (already examined in "A Magic Square Tribute to Dürer, 500+ Years After Melencolia I," and "Pan-Zigzag Magic Tori Magnify the "Dürer" Magic Square"). 

In the section reserved for correspondence at the end of the post "A Magic Square Tribute to Dürer, 500+ Years After Melencolia I," I have recently received some interesting comments from Rob Sellars. Rob looks at Dürer's engraving from a Judaic point of view and describes the bat-like animal (at the top left) as a flying chimera which has a combination of the Tinshemet features of the "flying waterfowl and the earth mole." Rob's description has made me look harder at this beast, and in doing so, I have noticed some aspects that explain the very essence of "Melencolia I."

 

The Historical Context

The year 1514 CE came during a turbulent historical period, just three years before the Protestant Reformation, and the seemingly endless wars of religion which would follow. When Albrecht Dürer created "Melencolia I," he was expressing the philosophical, scientific, and humanist ideas of fifteenth-century Italy, and thus contributing to the beginning of a new phase of the Renaissance. Dürer was one of the first artists of Northern Europe to understand the importance of the Greek classics, and particularly the ideas of Plato and Socrates. The Renaissance idea was revolutionary, as it suggested that everyone was created "in imago Dei," in the image of God, and was capable of developing himself, or herself, to participate in the creation of the universe. This idea was now being gradually transmitted to all classes of society, thanks to the invention of the printing press; but it required a metaphorical language which could be deciphered by all, especially in a largely illiterate population. Although most of Dürer’s prints were intended for this wide public, his three master engravings (“Meisterstiche”), which include "Melencolia I," were aimed instead at a more discerning circle of fellow humanists and artists. The messages were more intellectual, using subtle symbols that would not be evident for common men, but could be decrypted by those initiated in the art.

The Metamorphosis of the Flying Creature

Detail of the bat-like beast at the top left-hand side of "Melencolia I" which was engraved by Albrecht Dürer in 1514

At first sight, the cartouche at the top left-hand side of Dürer's "Melencolia I" seems to be a flying bat, bearing the title of the engraving on its open wings. The length and thickness of the tail both look oversized, but we can suppose that Dürer was using his artistic licence to amplify the visual impact of the swooping beast. Nearly all species of bats have tails, even if most (if not all) of these, are shorter and thinner than the one that Dürer has depicted.

But looking again with more attention, we can see that, quite weirdly, the body of the animal is placed above its wings, which is impossible unless the bat is flying upside-down! Closer examination suggests that this is not the case, as the mouth and eyes of the beast are clearly those of an animal with an upright head. All the same, we might well ask where the hind feet are, and how the creature can possibly make a safe landing without these!

Looking once again more closely, we can see another, even more troubling detail, in that the “wings,” which carry the title of the engraving, are in fact two large strips of ragged skin, ripped outwards from the belly, as if the animal has disembowelled itself!

Judging from the thickness of its tail and the form of its head, the airborne creature was initially a rat before it began its painful metamorphosis. It has since carried out an auto-mutilation, and is now showing its inner melancholy to the outer world, but at the same time flying free with its hard-earned wings!

Symbolically, the cartouche is telling us that ""Melencolia I" is a painful metamorphosis which precedes a liberating "Renaissance!""

How Melancholy leads to Renaissance

During 1514 CE the artist's mother, Barbara Dürer (née Holper), passed away, or “died hard” as he described it, and we can therefore suppose that Dürer’s grief would have been a strong catalyst of the very melancholic atmosphere depicted in his “Melencolia I.” The melancholy, referred to in the title of the engraving, is illustrated by an extraordinary collection of symbols that fill the scene. Some of these are tools associated with craft and carpentry. Others are objects and instruments that refer to alchemy, geometry or mathematics. In addition to the bat-like beast, the sky also contains what might be a moonbow and a comet. Further symbols include a putto seated on a millstone, and a robust winged person, also seated, which could well be an allegorical self-portrait. These, and many other symbols, are the object of multiple interpretations by various authors. Some scholars consider the engraving to be an allegory, which can be interpreted through the correct comprehension of the symbols, while others think that the ambiguity is intentional, and designed to resist complete interpretation. I tend to agree with the latter point of view, and think that the confusion symbolises the unfinished studies and works of the main melancholic figure; an apprentice angel, who believes that despite his worldly efforts, he lacks inspiration, and is not making sufficient progress.

Notwithstanding the melancholy that reigns, there is still hope: The 4 x 4 magic square, for example, has the same dimension as Agrippa's Jupiter square, a talisman that supposedly counters melancholy. The intent expression of the main winged person suggests a determination to overcome his doubts, and transcend the obstacles that continue to block his progression. Positive symbols of a resurrection or "Renaissance" are also plainly visible, not only in the hard-earned wings of the flying creature, but also in the growing wings that Dürer gives himself in his portrayal as the apprentice angel.

"Melencolia I" engraved by Albrecht Dürer in 1514

On page 171 of his book entitled "The Life and Art of Albrecht Dürer," Erwin Panofsky considers that "Melencolia I" is the spiritual self-portrait of the artist. There is indeed much resemblance between the features of the apprentice angel, and those of the engraver in previous self-portraits.

Dürer had already adopted a striking religious pose in his last declared self-portrait of 1500 CE, giving himself a strong resemblance to Christ by respecting the iconic pictorial conventions of the time. In other presumed self-portraits, (but not declared as such), Dürer had also presented himself in a Christic manner; in his c.1493 "Christ as a Man of Sorrows;" and in his 1503 "Head of the Dead Christ." What is more, Dürer inserted his self-portraits in altarpieces; in 1506 for the San Bartolomeo church in Venice ("Feast of Rose Garlands"); in 1509 for the Dominican Church in Frankfurt ("Heller Altarpiece"); and in 1511 for a Chapel in Nuremberg's "House of Twelve Brothers" ("Landauer Altarpiece" or "The Adoration of the Trinity"). Thus Dürer was already a master of religious self-portraiture when he engraved "Melencolia I" in 1514, and he might well have continued in the same manner. But this time, probably because the theological, philosophical and humanistic ideas of the Renaissance were not only spiritually, but also intellectually inspiring, he went even further, and gave himself wings!

Acknowledgement

Passages of "The Historical Context" are inspired by the writings of Bonnie James, in her excellent article "Albrecht Dürer: The Search for the Beautiful In a Time of Trials" (Fidelio Volume 14, Number 3, Fall 2005), a publication of the Schiller Institute.  

Latest Development

After reading this article, Miguel Angel Amela (who like me, is not only interested in magic squares, but also in "Melencolia I") sent me his thanks by email, and enclosed "a paper of 2020 about a painful love triangle..." His paper is entitled "A Hidden Love Story" and interprets the "portrait of a young woman with her hair done up," which was first painted by Albrecht Dürer in 1497, and then reproduced in an engraving by Wenceslaus Hollar, almost 150 years later in 1646. Miguel's story is captivating, and I wish to thank him for kindly authorising me to publish it here.

Read more ...